\( \newcommand{\matr}[1] {\mathbf{#1}} \newcommand{\vertbar} {\rule[-1ex]{0.5pt}{2.5ex}} \newcommand{\horzbar} {\rule[.5ex]{2.5ex}{0.5pt}} \newcommand{\E} {\mathrm{E}} \)
deepdream of a sidewalk
Show Question
\( \newcommand{\cat}[1] {\mathrm{#1}} \newcommand{\catobj}[1] {\operatorname{Obj}(\mathrm{#1})} \newcommand{\cathom}[1] {\operatorname{Hom}_{\cat{#1}}} \newcommand{\multiBetaReduction}[0] {\twoheadrightarrow_{\beta}} \newcommand{\betaReduction}[0] {\rightarrow_{\beta}} \newcommand{\betaEq}[0] {=_{\beta}} \newcommand{\string}[1] {\texttt{"}\mathtt{#1}\texttt{"}} \newcommand{\symbolq}[1] {\texttt{`}\mathtt{#1}\texttt{'}} \newcommand{\groupMul}[1] { \cdot_{\small{#1}}} \newcommand{\inv}[1] {#1^{-1} } \)
Math and science::Algebra::Aluffi

Normal subgroup

Normal subgroup

Let \( G \) be a group and \( N \) a subgroup of \( G \).

\( N \) is said to be normal iff:

\[ \forall n \in N, \forall g \in G, \; gng^{-1} \in N \]

Or equivalently:

\[ N \text{ is normal } \iff \forall g \in G, \; gN = Ng \]

\( gN \) is syntax for \( \{ a : a = gn \text{ for some } n \in N \} \), and \( gN = Ng \) does not imply commutativity.


Example

Abelian normal subgroup

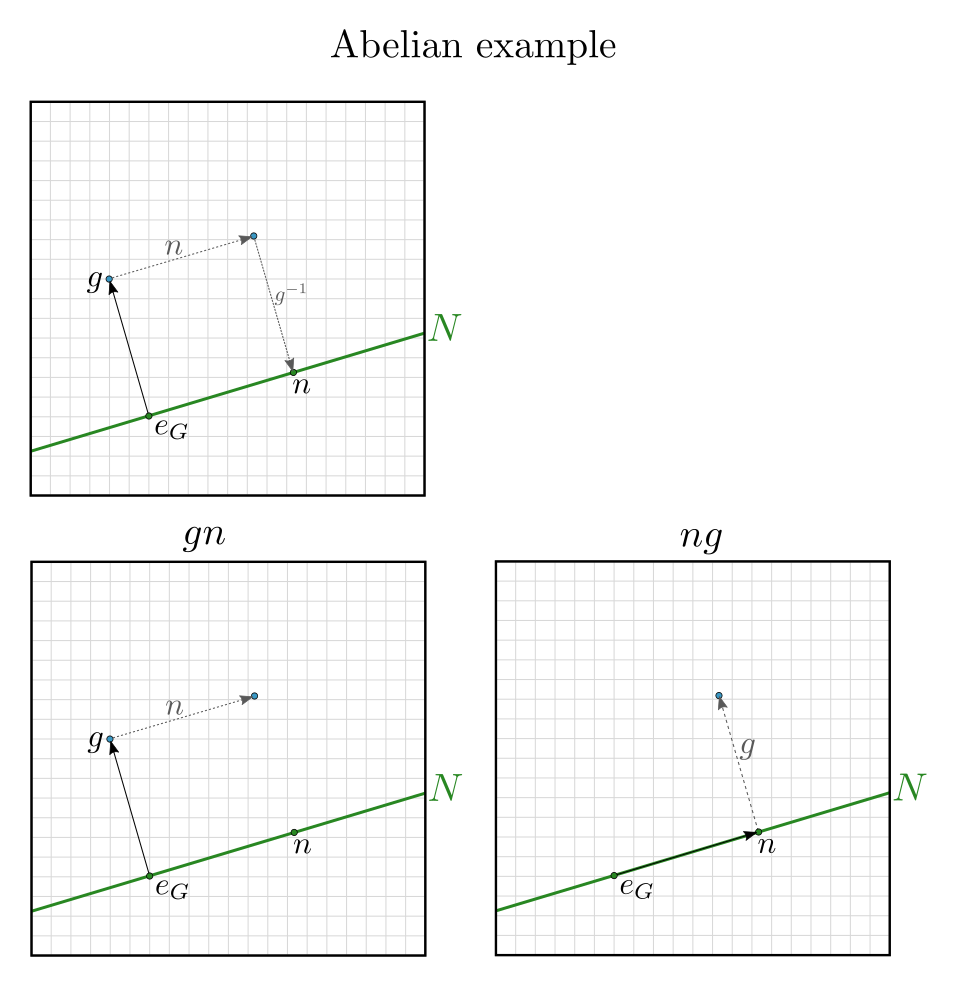
Note how in the Abelian case, \( gng^{-1} = n \), which is a stronger result than \( gng^{-1} \in N \).

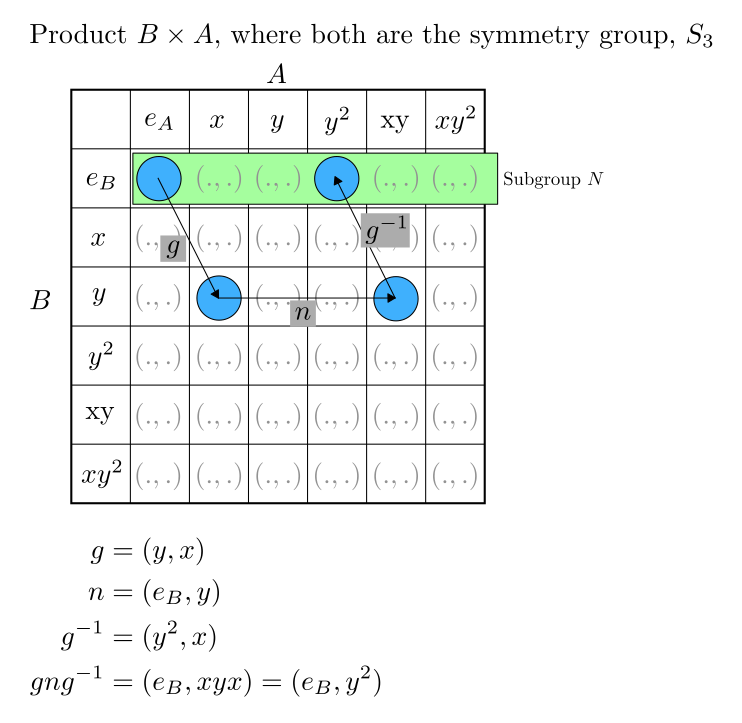
Non-abelian normal subgroup

Products have non-abelian normal subgroups.