\( \newcommand{\matr}[1] {\mathbf{#1}} \newcommand{\vertbar} {\rule[-1ex]{0.5pt}{2.5ex}} \newcommand{\horzbar} {\rule[.5ex]{2.5ex}{0.5pt}} \newcommand{\E} {\mathrm{E}} \)
deepdream of a sidewalk
Show Question
\( \newcommand{\cat}[1] {\mathrm{#1}} \newcommand{\catobj}[1] {\operatorname{Obj}(\mathrm{#1})} \newcommand{\cathom}[1] {\operatorname{Hom}_{\cat{#1}}} \newcommand{\multiBetaReduction}[0] {\twoheadrightarrow_{\beta}} \newcommand{\betaReduction}[0] {\rightarrow_{\beta}} \newcommand{\betaEq}[0] {=_{\beta}} \newcommand{\string}[1] {\texttt{"}\mathtt{#1}\texttt{"}} \newcommand{\symbolq}[1] {\texttt{`}\mathtt{#1}\texttt{'}} \newcommand{\groupMul}[1] { \cdot_{\small{#1}}} \newcommand{\inv}[1] {#1^{-1} } \)
Math and science::Algebra::Aluffi

Groups. Kernel.

Kernel of a group homomorphism

Let \( \varphi : G \to G' \) be a group homomorphism from group \( G \) to group \( G' \). The kernel of \( \varphi \) is the set of elements of \( G \) that map to \( e_{G'} \) through \( \varphi \).

The kernel of \( \varphi \) is denoted as \( \operatorname{ker} \varphi \).


In other words, \( \varphi \) is the preimage of \( \{ e_{G'} \} \) through \( \varphi \).

\[ \operatorname{ker} \varphi = \varphi^{-1}(\{ e_{G'} \}) \]

From kernel to (equal) cosets

For the below discussion, it's worth remembering that kernels form subgroups, and these subgroups are normal.

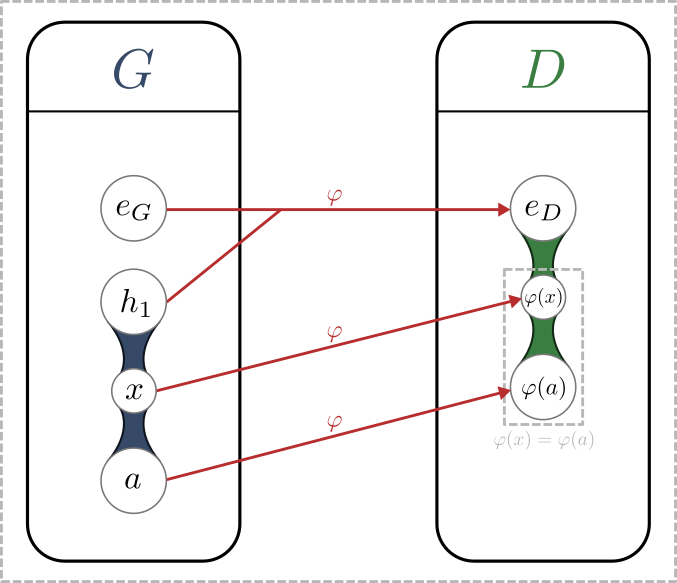
Let \( \varphi : G \to D \) be a group homomorphism. Consider the case if \( \operatorname{ker} \varphi \) has 2 elements, \( (e_G, h_1) \). The fact that \( \varphi \) is a group homomorphism introduces some strong requirements on other elements of \( G \). Let \( a \in G \) be an element not in \( \operatorname{ker}(\varphi) \). Then consider:

\[ \begin{align*} a \cdot e_G &= a \\ a \cdot h_1 &= x \\ h_1 \cdot a \; \, &= \; ? \\ \end{align*} \]

Note that \( a h_1 = h_1 a \). This commutativity is required so that \( e_D x = x e_D \) in the group \( D \).

In the group \( G \), \( e_G \) and \( h_1 \) are distinct elements, and so \( a \) and \( x \) must also be distinct elements, by the nature of groups. As \( D \) is also a group and \( \varphi \) a group homomorphism, \( a \) and \( x \) must map through \( \varphi \) to the same element in \( D \). This is required to satisfy the requirement of group homomorphisms:

\[ \forall w,v \in G, \; \varphi( w \groupMul{G} v) = \varphi(w) \groupMul{D} \varphi(v) \]

This means that for any \( d \in D \), the preimage of \( d \) through \( \varphi \), \( \operatorname{im} \varphi( \{ d \} \), must have the same number of elements as \( \operatorname{ker} \varphi \)! We can use these preimages to form the blocks in a partition and the resulting equivalence relation is exactly the one corresponding to the quotient \( G \!/ \operatorname{ker}(\varphi) \).

Below is a visualization of this idea.

Source

Aluffi p80