Math and science::Topology

Metric space

Metric

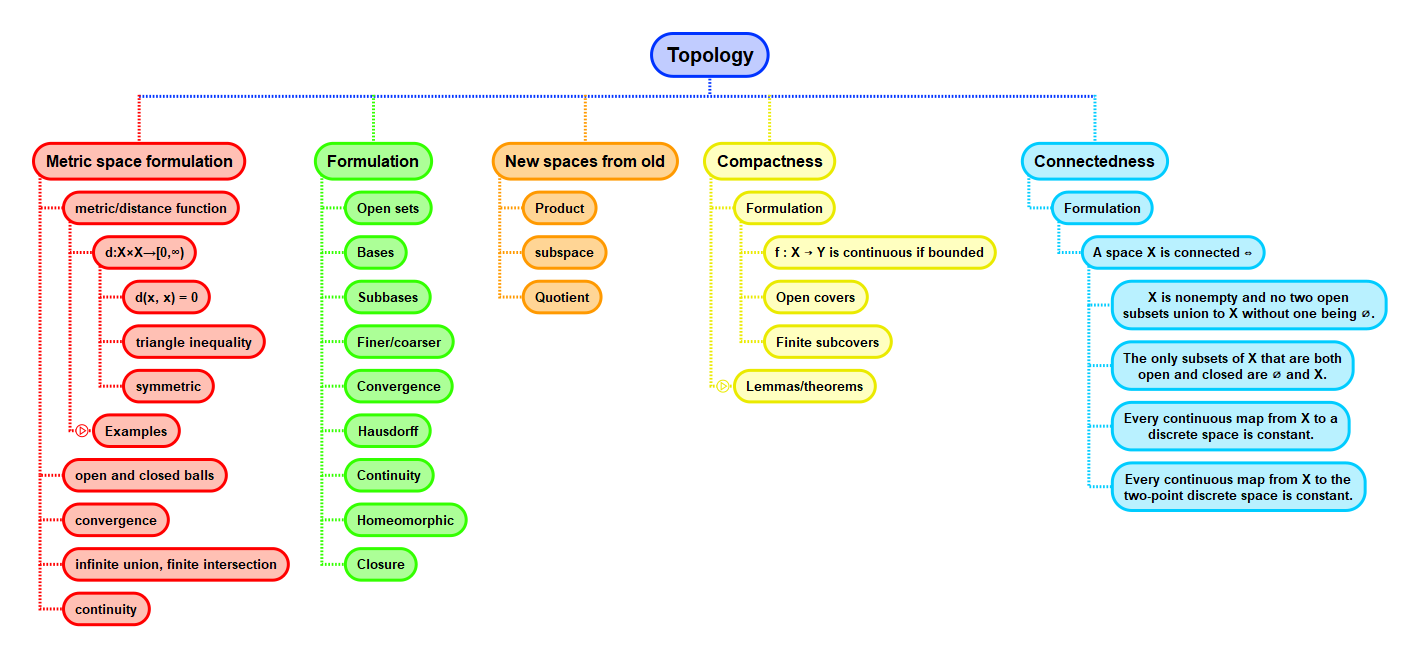
A metric \( d \) on a set \( X \) is a function \( d : X \times X \to [0, \infty) \) with the following three properties:

What is this called?: \( d(x, y) = 0 \iff x = y, \text{ for all } x, y \in X \)
Triangle inequality: \( d(x, y) + d(y, z) \ge d(x, z), \text{ for all } x, y, z \in X \)
Symmetry: \( d(x, y) = d(y, x), \text{ for all } x, y \in X \)

A metric space is a set together with a metric on it, or more formally, a pair \( (X, d) \) where \( X \) is a set and \( d \) is a metric on \( X \).

Example

Some interesting metrics

1. \( d_1, d_2, d_\infty \) (instances of \( d_p \)) on \( \mathbb{R}^n \)

For all \( x = (x_1, ..., x_n) \) and \( y = (y_1, ..., y_n) \) in \( \mathbb{R}^n \) (or any subset thereof):

The Euclidean metric, \( d_2 \) on \( \mathbb{R}^n \) is given by: \( d_2(x, y) = (\sum_{i=1}^{n} (x_i - y_i)^2)^{\frac{1}{2}} \)
\( d_1 \) is given by: \( d_1(x, y) = \sum_{i=1}^{n}|x_i - y_i| \)
\( d_\infty \) by: \( d_\infty(x, y) = max_{1 \le i \le n}|x_i - y_i| \)

All these metrics are particular cases of the \( d_p \) metric.

2. \( d_1, d_2, d_\infty \) (instances of \( d_p \)) on \( C[a, b] \))

All \( d_p \) metrics have parallels over function spaces. \( C[a, b] \) is the set of continuous functions \( [a,b] \to \mathbb{R} \). Three interesting metrics on \( C[a, b] \) are given by:

\[ d_1(f, g) = \int_{a}^{b} |f(t) - g(t)|dt \]

\[ d_2(f, g) = \left( \int_{a}^{b} |f(t) - g(t)|dt \right)^{\frac{1}{2}} \]

\[ d_\infty(f, g) = \sup_{a \le t \le b}|f(t) - g(t)| \]

3. Hamming metric

With \( A \) being any set, and \( x, y \in A^n \), the Hamming metric \( d \) on \( A \) is given by

\( d(x, y) = |\{i \in \{1, ..., n\} : x_i \ne y_i \}| \)